There are many applications for the arc length of functions. I came across it when I needed to calculate the length of a density function in an interval . The formula reads
Do you grasp how this formula arises? No? Let me show you!
I imagined how I zoom into a function until single points become visisble. Actually, this is not possible from a mathematical point of view – but let’s try for simplicity’s sake 🙂 The following sketch shows what I mean:
As you see, the horizontal distance is denoted by while the vertical distance is denoted by
.
can be expressed in terms of
and the derivative
:
At the same time, Pythagoras’ theorem holds and allows us to calculate the infinitesimal distance . I also applied some manipulations to the expression.
Adding these endlessly, small sections of the line together is done via a sum. Just write the integral sign in front of the formula.
Done. 😉





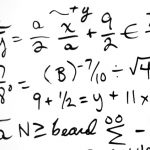
Leave a Reply